TRIGONOMETRY-(RATIOS,IDENTITIES AND HEIGHTS AND DISTANCES)

TRIGONOMETRY

(RATIOS, IDENTITIES AND HEIGHTS AND DISTANCES)

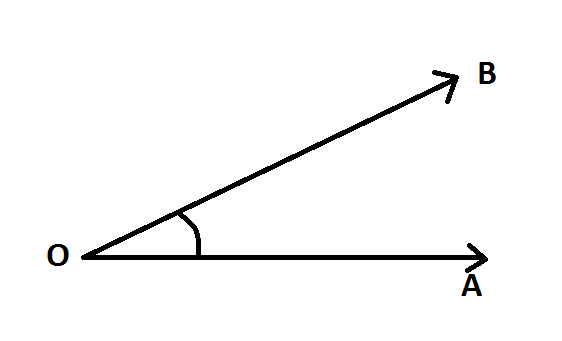
Trigonometry deals with the study of triangles, the measurement of their sides, angles and the various relations which exist between them.

Angle: An angle, in trigonometry, is formed by the rotation of a ray in a plane around the end point.

In the above figure, OA is the initial position and OB is the final position of the ray.

Positive and Negative Angles:  If the rotation of the ray OA about 'O' is in the anticlockwise direction, then the angle AOB is positive and if the ray rotates in the clockwise direction, the angle made is considered as negative.

Quadrants:  The concept of quadrants was discussed in the chapter Co-ordinate Geometry in detail. We now summarize this concept diagrammatically.

Systems of Measurement of Angles: 

Initially, we understand the division of a right angle.
1 right angle=90^o (ninety degrees)
1^o =60' (Sixty minutes)
1'=60" (Sixty seconds)

1. In Sexagesimal system.

1 full revolution = 360^o   (360 degrees)
1^o                  = 60'        (60 minutes)
1'                     = 60"        (60 seconds)
1 right angle     = Quarter revolution = 90^o

2. In Centesimal System:

1 full revolution = 400^g   (400 grades)
1 right angle     = quarter revolution
                       =  100 grades
1 grade           = 100'        (100 minutes)
1'                    = 100"       (100 seconds)

3. In Radian Measure:

This system of measurement is also called circular system.

Radian:    It is the angle subtended at the center of a circle by an arc whose length is equal to the radius of
               the circle. The radian is a constant angle.

The angle AOB is taken as the unit of circular system. This angle is called a Radian and is denoted by 1^o.

Relation between Sexagesimal and Circular system:

1. π radians = 2 right angles =180^o 

2. 1^{0    =}180^o/ π and 1⁰=  π/180  radians

3.   1⁰= 57^o 17’ 44.8”

Radian measures of some common angles are given below.

Length of an arc of an angle:  If "l' is the length of the arc of a circle  of radius "r" and if this arc subtends an angle "θ"  (in radians) at the center of the circle, then l=rθ.

Note: "l" and "r" must be in the same units and  θ must be in radians.

Trigonometric Ratios:   There are six possible ratios among the three sides of a right angled triangle. These six ratios are called trigonometric ratios or circular functions. They are defined as follows w.r.t the right angled triangle shown.

Sine of the angle θ or briefly sinθ

=Perpendicular/Hypotenuse=PM/OP

Cosine of the angle θ or briefly cosθ

=Base/Hypotenuse=OM/OP

Tangent of the angle θ or briefly tanθ

=Perpendicular/Base=PM/OM

Cosecant of the angle θ or briefly cosecθ

=Hypotenuse/Perpendicular=OP/PM

Secant of the angle θ or briefly secθ

=Hypotenuse/Base=OP/OM

Co tangent of the angle θ or briefly cotθ

=Base/Perpendicular=OM/PM

Relations between trigonometric ratios:

1. sinθ. Cosecθ =1  ==> sinθ=1/Cosecθ or Cosecθ=1/sinθ
2. Cosθ. secθ =1  ==> cosθ=1/secθ or secθ=1/cosθ
3. tanθ. cotθ =1  ==> tanθ=1/cotθ or cotθ=1/tanθ
4. tanθ= sinθ/cosθ   
5. Cotθ = cosθ/sinθ

Also remember,

1. Sin² θ+Cos² θ = 1  èsin² θ= 1-cos² θ or cos² θ = 1-sin² θ
2. Sec ² θ-tan² θ = 1 è tan² θ=sec² θ-1 or sec² θ= 1+tan² θ
3. Cosec² θ-cot² θ = 1 è cosec² θ = 1+cot² θ or cot² θ=cosec² θ-1

Note 1: All the above identities are independent of the angle. Whatever may be the angle. the above relations are true.

Note 2: =(sin θ)² is represented as Sin² θ and so on.

Signs of Trigonometric Ratios:

1. If  θ lies in the first quadrant (0<θ<π/2), all the trigonometric ratios are positive.
2. If  θ lies in the Second quadrant (π/2<θ<π), only Sinθ and cosecθ are positive and the rest of the ratios are negative.
3. If  θ lies in the Third quadrant (π<θ<3 span="">π/2), only tanθ and cotθ are positive and the rest of the ratios are negative.
4. If  θ lies in the Fourth quadrant (3π/2<θ<2 span="">π), only cosθ and secθ are positive and the rest of the ratios are negative.

The signs of the trigonometric ratios can be remembered with the help of the diagram given below.

The maximum and minimum values of trigonometric Ratios:

1. The sine and cosine of an angle can never be less than -1 and cannot be greater than +1 
2. The secant and cosecant of an angle will not have nay value between -1 and +1.
3. The tangent and co tangent of an angle can take any real value.

Values of Trigonometric functions of some standard angles:

Periodic Functions

A function f(x) is said to be periodic if there exists a real k > 0 such that f(k+x)=f(x). The least possible value of k is the period of the function. i.e., if by changing x to x+k (k being least positive constant), the function f(x) remains unaltered, then f(x) is called a periodic function and k the period.

The values of sine, cosine, secant and cosecant functions repeat after an interval of 2π. Hence 2π is the period of these functions.

sin(x+2π) = sinx for all x belongs to R;
cos9x+2π) = cosx  and so on.
Since tan(x+π)=tanx and cot(x+π)=cot x, π being the least positive integer, π is the period of tan x and cot x.

To summarise,

1. 2π is the period of sin x, cos x, cosec x and sec x.
2. π is the period of tan x and cot x.
3. Period of functions of the form sin ax, cos(ax+b) is 2π/a;  period of 4 sin 5x = 2π/5.
4. Period of function of form  f(x)+g(x)/h(x)+k(x) is the L.C.M. of the period of f(x), g(x), h(x) and k(x).

Maximum and minimum values of (acosx+bsinx+c):

Whatever be the value of x, the expression a cos x + b sin x + c lies between c±sqrt(a^2+b^2) i.e.,; the minimum value is c-sqrt(a^2+b^2) and the maximum is c+sqrt(a^2+b^2).

Heights and Distances:

Let AB be a vertical drawn from B to A  to meet the horizontal line drawn from O (which is at lower level than B) and let BO' be the horizontal drawn through B (i.e., BO' is parallel to OA)

Then AOB is called the angle of elevation of point B as seen from point O. O'BO is the angle of depression of point O as seen from point B. As can be readily seen, since AO is parallel to BO' 

α = β

i.e., Angle of elevation = Angle of depression.

Using trigonometry ratios on angles of elevation and depression, we can find out heights and distances as seen in examples given part of this chapter.

Worked out examples:

1. The angle given below are in degrees. Give their corresponding radian measure.

(a) 67 1/2 degrees        (b)54 degrees

Answer: (a) 67 1/2 degrees = 67 1/2 * π/180 (Since 1 degree = π/180)

                                                 =135/2 * π/180

                                                 =3π/8 radians

               (b) 54 degrees = 54 * π/180

                                             =3 π/10 radians.

----------------------------------------------------------------------------------------------------------------------------------

2. The angles given below are in radians. Give their corresponding values in degrees.

(a) 5 π/18      (b)7π/5

Answer: (a) 5π/18=5π/18 * 180/π

                                        =50 degrees

                     (b)7π/15 = 7π/5 * 180/π

                                        =252.

----------------------------------------------------------------------------------------------------------------------------------

3. Find each interior angle of a regular octagon.

Answer: A regular octagon has eight sides which are equal.

Sum of 8 exterior angles = 360 degrees

Each exterior  angle =360/8=45 degrees

Then each interior angle= 180 degrees-45 degrees =135 degrees.

----------------------------------------------------------------------------------------------------------------------------------

4. Find the ratio of lengths of arcs of two circles with same radius which subtend angles of 15 degrees and 60 degrees respectively at the center.

Answer: θ1=15 degrees=π/12 radians

                    θ2=60 degrees=π/3 radians 

As the circles have same radius r1=r2

The length of an arc "l" of a circl having a radius "r", subtending an angle of 'θ' (in radians) at the center is given by l=rθ. 

for the two arcs given, l1=r1θ1 and l2=r2θ2

==> l1/l2=r1θ1/r2θ2=π/12 /π/3=1/4

l1:l2=1:4.

----------------------------------------------------------------------------------------------------------------------------------

5. A horse tied to post by a rope 105m long. if the horse moves always keeping the rope tight in a fixed direction, What distance has it covered when the rope moves by an angle 54 degrees?

Answer:  The distance covered by the horse is the length of the arc of a circle having radius 105 m , the arc subtending an angle of 54 degrees at the center. 

r= 105 m
θ=54 degrees=54*π/180=3π/10 radians
As l=rθ
l=105 * (3/10)*(22/7)=99m

----------------------------------------------------------------------------------------------------------------------------------

6. If sec θ + tan θ =5, sin θ (θ is an acute angle)

Answer: Given  sec θ + tan θ =5 ---> (1)
==> sec θ -tan θ=1/5 --->(2)
Adding (1) and (2) we get
==> 2 sec θ = 13/5
sin θ=12/13 
----------------------------------------------------------------------------------------------------------------------------------

7. If α + β =π/2, Sin α =sqrt(3)/2 and α,β are acute, then find the value of sqrt(cosα cosβ) 

Answer: Given  α + β =π/2 Sin α =sqrt(3)/2
==> α=π/3(α is acute), then
β =π/2- π/3=π/6
now consider sqrt(cosα cosβ) =sqrt(1/2.sqrt(3)/2)=1/2(3)^1/4.

----------------------------------------------------------------------------------------------------------------------------------

8. Find the value of [(sin 750 deg)(cot 1020 deg)]-[cos(-600)deg tan 930 deg].

Answer: sin 750 deg. cot 1020 deg-cos(-600)deg tan 930 deg
=sin 750 deg. cot 1020 deg-cos(600)deg tan 930 deg
Since cos(-θ)=cos θ
=sin(2.360+30)deg cot(3.360-60)deg-cos(2.360-120)deg tan(2.360+210)deg
=-1/2*1/sqrt(3) - (-1/2)*1/Sqrt(3)
=-1/2sqrt(3)+1/2sqrt(3)=0
---------------------------------------------------------------------------------------------------------------------------------

9. If A+B=135 degrees, then prove that (cot A+1)(Cot B+1)=2?

Answer:  Given A+B =135 degrees
Taking cot on both sides we get 
 cot(A+B)=Cot(135)
cot A. cot B-1/(cot B+Cot A) = -1
cot A. cot B-1=-cot B -cot A
cot A+cot B+cot A * cot B =1
1+cot A+ cot B + cot A. cot B = 1 + 1
(1+cot A)(1+cot B)=2

----------------------------------------------------------------------------------------------------------------------------------

10. find the value of cos 671/2 deg + sin 67 1/2 deg/(cos 67 1/2 deg- sin 67 1/2 deg)

Answer: Dividing numerator and denominator by cos 67 1/2 deg we get 1+ tan 67 1/2 deg/(1-tan 67 1/2 deg)
=tan 45 deg +tan 67 1/2 deg / (1-tan 45 deg tan 67 1/2 deg)
=tan(45 deg+ 67 1/2 deg)= tan (112 1/2 deg)
=tan (90+ 22 1/2 deg)= -cot 22 1/2 deg

----------------------------------------------------------------------------------------------------------------------------------

11. find the value of 

a. cos 75 deg     b.tan 67 1/2 deg

Answer: a.cos 75 deg= cos (45 + 30) deg
= cos 45 deg cos 30 deg - sin 45 deg sin 30 deg
=1/sqrt(2) . sqrt(3)/2-1/sqrt(2). 1/2=sqrt(3)-1/2sqrt(2)

b. Let A=67 1/2 deg then 2A =135 deg
We know that tan A = sinA/cos A
=sqrt(1-cos2A/1+cos2A)

tan 67 1/2 deg = sqrt(1-cos135/1+cos135)

=sqrt(1+1/sqrt(2)/1-1/sqrt(2))=sqrt(sqrt(2)+1/sqrt(2)-1)
=sqrt((sqrt(2)=1)^2/2-1)=sqrt(2)+1

----------------------------------------------------------------------------------------------------------------------------------

12. Find the maximum and minimum values of 16 cos x + 12 sin x - 9

Answer: The maximum and minimum values of    a sinx + b cos x + c are given by  c+or - sqrt(a^2-b^2)

Maximum value= -9 + sqrt(16^2+12^2)
                           = -9 + sqrt(256+144)= -9 + 20 =11

Minimum value= -9 - sqrt(16^2+12^2)
                           = -9 - sqrt(256+144)= -9 - 20 = -29

Therefore the maximum and minimum values are 11 and -29.

----------------------------------------------------------------------------------------------------------------------------------

13. If 1 + cos θ/1- cos θ  = b^2/a^2, then find the value of sec θ  in terms of a and b 

Answer: Given 1+cos θ/1-cosθ =b^2/a^2

a^2+a^2 cos θ= b^2 - b^2 cos θ
(a^2+b^2)cos θ=b^2-a^2
cos θ= b^2-a^2/(a^2+b^2)

sec θ= 1/cosθ =a^2+b^2/b^2-a^2

----------------------------------------------------------------------------------------------------------------------------------

14. if x=a sec ^n θ and y =b tan ^m θ eliminate θ to get the relation between x and y?

Answer: 

Given x = a sec^n θ and y= b tan^m θ

x/a=sec^n θ and y/b = tan^m θ

(x/a)^1/n= sec θ  and (y/b)^1/m = tan θ

we know that sec^2 θ-tan^2 θ=1

(x/a)2/n-(y/b)2/m=1

----------------------------------------------------------------------------------------------------------------------------------

15.The top of a flag pole makes an angle of 30 deg at a point on the ground. If the distance between the point of observation and the foot of the pole is 150 m , find the height of the flap pole.

Answer:    Consider the right angled triangle ABC, Where AB represents the height of the pole and C is the point of observation.

Given BC = 150 m
tan 30 deg = AB/BC=h/150
h= 150(1/sqrt(3))=50sqrt(3)

Therefore the height of flag pole = 50sqrt(3)

----------------------------------------------------------------------------------------------------------------------------------
16.The upper part of a pole is broken over by the wind makes an angle 45 deg with the ground. the distance from the foot of the tree to the point where the top  of the pole touches the ground is 30 m. What was the (unbroken) height of the pole?

Answer:      

 
From the figure, AB + AC represents the height of the pole and AC is the broken part of the pole.

tan 45 deg = AB/BC= AB/30
AB=30 m and cos 45 deg = BC/AC
1/sqrt(2)=30/AC
AC= 30sqrt(2)
The height of the pole AB+AC=30+30sqrt(2) m
=30(1+ sqrt(2)) m
----------------------------------------------------------------------------------------------------------------------------------


17.A man wishes to find the height of the building which stands on a horizontal plane. At a point on the plane, he finds the angle of elevation of the top of the building to be 60 deg  and on moving 20m along the same line away from the building, he finds the angle of elevation to be 30 deg . Find the height of the building?

Answer:  

Let AB be the height of building. 

From ABC, tan 60 deg = AB/BC
BC= h/sqrt(3)  eqn (1)

From triangle ABD, tan 30 deg = AB/BD
=AB/(BC+CD)
BC + 20 =AB(sqrt(3))
BC= sqrt(3) h -20 eqn (2)

From (1) and (2) we have h/sqrt(3) =sqrt(3)h-20
h= 3h-20sqrt(3)
2h=20sqrt(3)
h=10sqrt(3).

----------------------------------------------------------------------------------------------------------------------------------

18.A flag post stands on the top of a tower. The angles of elevation of the top of the tower and the flag post  from a  point 108 m  from the foot of the tower are found to be 30 deg and 60 deg respectively. What is the height of the flat post.

Answer:  

Let BC represent the tower, AB the flag post and D be the point of observation. 
Given CD=108 m
From triangle BCD, tan 30 deg = BC/DC
 BC = 1/sqrt(3)* 180 = 60 sqrt(3) m
From triangle ACD, tan 60 deg = AC/CD
=(AB+BC)/CD
AB+ BC = 180 sqrt(3)
AB = 180sqrt(3) - 60sqrt(3)
       = 120sqrt(3) m
----------------------------------------------------------------------------------------------------------------------------------

19.From the top of tower 150 m high, the angles of depression of the top and bottom of a building are observed to be 30 deg and 60 deg respectively. Find the height of the building.

Answer:  

Let AB be the tower and CD be the building 

Given AB =150 m
In triangle AEC, tan 30 deg = AE/EC

CE = AE sqrt(3) and in 
Triangle ABD, tan 60 deg = AB/BD

BD= AB 1/sqrt(3)
But CE= BD and AB= AE +EB
AE sqrt(3)= AB(1/sqrt(3))
3AE = AB =150 
AE = 50 m
CD = BE= 150 - 50 
=100 m
----------------------------------------------------------------------------------------------------------------------------------


20.Two buildings of equal heights stand on either side of a road. At a point on the road in between the buildings the angles of elevation of the top of the two buildings are observed to be 45 deg and 60 deg . If the distance between the building is 150 m, then what is the height of the building?
Answer:  

Let AB,CD be the two buildings each of height  h and E be the point of observation.
Let DE = x , the BE = 150 -x 
Since BD = 150 m

In triangle CDE, tan 45 deg = CD/DE

h = x eqn(1)

From triangle ABE, tan 60 deg = AB/BE = h/150-x
150-x=h/sqrt(3) = x=150 -h/sqrt(3) eqn(2)

From (1) and (2) we get h = 150-h/sqrt(3)
h = 150sqrt(3)/sqrt(3) + 1= 75(3-sqrt(3)) m.

----------------------------------------------------------------------------------------------------------------------------------

QUESTIONS & ANSWERS

1. The value of 6π/5 in sexagesimal measure is 

(1)144 deg      (2)216 deg     (3)240 deg   (4)120 deg

Answer:  We know that π = 180 deg

 6/5 * 180 deg

= 6*36 deg

= 216 deg.

----------------------------------------------------------------------------------------------------------------------------------

2. The value of 72 deg in circular measure is 

(1)2π/5         (2)4π/5             (3)80 deg                        (4)160 deg

Answer: We know that 1 deg = π/180 radians

72 deg= 72*π/180=2π/5.

----------------------------------------------------------------------------------------------------------------------------------

3. The radius of a circle is 22 cm. Angle subtended by its arc of length 110 cms is

(1)900 deg/π         (2)π deg/36             (3)36/π deg                       (4)π deg/900

Answer: Given radius r= 22 cm and length l= 110 cm
We know l = rθ, 110 = 22 θ 

θ = 110/22=5

Since θ=π/180 deg

5 deg = π/180 deg

900 deg =π

900 deg/π

Angle is 900 deg/π

----------------------------------------------------------------------------------------------------------------------------------

4 A wheel makes 12 revolutions per hour. The radians it turns through in 20 minutes is 

(1)8π         (2)16π             (3)24π                (4)32π 

Answer:  We know that 1 revolution = 360 deg

Given 1 hour =12 revolutions

60 minutes = 12 revolutions

1 minute = 12/60=1/5

Therefore 20 minutes = 20* 1/5 = 4 revolutions

Therefore it makes 4 revolutions in 20 minutes.

Since 1 revolution = 360 deg

         4 revolutions = 4* 360 deg

                               = 4 * 360* π/180 radians

                                         = 8π radians

----------------------------------------------------------------------------------------------------------------------------------

5.If θ is acute and cosec θ =17/8, then cot θ is

(1)8/15        (2)15/17             (3)15/8                (4)17/15 

Answer: Given cosec θ =17/8.

We know that cosec θ = 1/ sin θ 

sin θ = 8/17.

Applying Pythagoras theorem

AC^2 = AB^2+BC^2

17^2=8^2+BC^2

BC^2=289-64=225

BC=15.

Cos θ = 15/17.

Cot θ = cos θ/sin θ= 8/17/15/17=8/15.

----------------------------------------------------------------------------------------------------------------------------------

 6. sin 30 deg cos(π/3)+cos 30 deg* sqrt(3)/2=

(1)1         (2)1/2             (3)1/3                (4)sqrt(3) 

Answer:  sin 30 deg cos(π/3)+cos 30 deg* sqrt(3)/2

= 1/2*1/2+sqrt(3)/2* sqrt(3)/2

=1/4+3/4= 4/4 =1

----------------------------------------------------------------------------------------------------------------------------------

7. If 3 cos^2A=cos 60 deg + sin ^2 45, then sec^2 A=

(1)1         (2)1/2             (3)1/3                (4)sqrt(3) 

Answer:  Given 3cos ^2 A = cos 60 deg + sin ^2 45 deg
                                            = 1/2 + (1/sqrt(2))^2
                                            =1/2 + 1/2
                                3 cos ^2 A = 1
cos ^2 A = 1/3
Since sec θ = 1/ cos θ
Therefore Sec ^2 A = 1/1/3= 3
----------------------------------------------------------------------------------------------------------------------------------

8. 3(cos ^2 45 deg + sin ^2 45 deg) - 3(sin ^2 225 deg + cos ^4 225 deg)=

(1)4/3         (2)1/9             (3)3/4                (4)1/2

Answer:  3((1/sqrt(2))^2+(1/sqrt(2))^2)-3(sin ^2(180+45)+ cos ^4 (180+45))
3(1/2+1/2)-3(sin ^2 180 cos^2 45+cos^2 180 sin ^2 45+ cos^4 180 cos^4 45-sin ^4 180 sin ^4 45)
3(1)-3(0*1/2+(1)*1/2+1*1/4-0*1/4)
3-3(1/2+ 1/4)
=3-3(3/4)
=12-9/4
=3/4
----------------------------------------------------------------------------------------------------------------------------------

9. The value of sec 7π/4

(1)sqrt(2)         (2)1/sqrt(2)             (3)-1/sqrt(2)                (4)-sqrt(2)

Answer: sec 7π/4

=sec 7/4*180
=sec 315 deg
=1/cos 315 deg
=1/cos(360-45)
=1/(cos 360 cos 45 + sin 360 sin 45)
=1/(1*1/sqrt(2) + 0* 1/sqrt(2))
=1/(1/sqrt(2))
=sqrt(2)
----------------------------------------------------------------------------------------------------------------------------------